Content

• To step
• Tips

As a graph see a quadratic equation ax + bx + c , also which is written as a (x - h) + k, look like a smooth curve in a U-shape. We call this one parabola. Graphing a quadratic equation involves finding the vertex, the direction, and often the intersection points with the x-axis and the y-axis. In the case of the relatively simple quadratic equation, it may also be sufficient to enter a number of values ​​for x to indicate these points in the coordinate system, after which the parabola can be drawn. Continue to step 1 to get started.

To step

1. Determine what kind of second-degree equation you have. It can be written in two ways: the standard notation and the vertex notation (another way to write the square root formula). You can use both to create a graph of a quadratic equation, but the process is slightly different in each case. Most of the time you will encounter the standard shape, but it certainly doesn't hurt to learn to use both shapes. The two forms of a quadratic equation are:
   • The standard shape. The quadratic equation is noted as: f (x) = ax + bx + c where a, b, and c are real numbers and a is not equal to zero.
     • Two examples of standard quadratic equations: f (x) = x + 2x + 1 and f (x) = 9x + 10x -8.
   • The vertex shape. The quadratic equation is noted as: f (x) = a (x - h) + k where a, h, and k are real numbers and a is not equal to zero. This shape is called vertex because h and k refer directly to the top of your parabola at the point (h, k).
     • Two examples of vertex form equations are f (x) = 9 (x - 4) + 18 and -3 (x - 5) + 1
   • To make a graph of these equations, we will first determine the top (h, k) of the graph. In the standard equation you will find this via: h = -b / 2a and k = f (h), while this is already given in vertex form because h and k occur in the equation.
2. Determine your variables. To solve a quadratic equation it is usually necessary to determine the variables a, b, and c (or a, h, and k). A regular exercise will give you a second degree equation in the standard form, but the vertex notation may also occur.
   • For example: the standard function f (x) = 2x + 16x + 39. Here we have a = 2, b = 16, and c = 39.
   • In vertex notation: f (x) = 4 (x - 5) + 12. Here we have a = 4, h = 5, and k = 12.
3. Calculate h. In the vertex notation, the value of h is already given, but in the standard notation this value has yet to be calculated. Remember that with the standard equation holds: h = -b / 2a.
   • Example 1. (f (x) = 2x + 16x + 39), h = -b / 2a = -16/2 (2). By solving this we see that h = -4.
   • Example 2. (f (x) = 4 (x - 5) + 12), we immediately see that h = 5.
4. Calculate k. As with h, k is already known from vertex form equations. For equations in standard notation, remember that k = f (h). In other words, you can find k by replacing any variable x with the value of h.
   • We have seen for example 1 that h = -4. To find k, we solve this equation by filling in this value of h in the equation, for the variable x:
     • k = 2 (-4) + 16 (-4) + 39.
     • k = 2 (16) - 64 + 39.
     • k = 32 - 64 + 39 = 7
   • From example 2 we know that the value of k is equal to 12, without the need for any calculation.
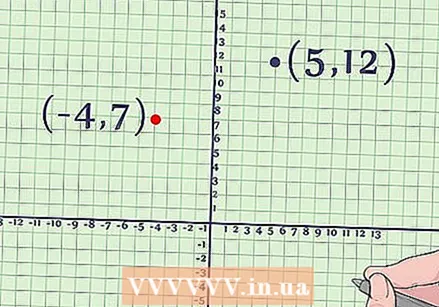5. Draw the top or bottom of the graph. The apex or valley of your parabola is the point (h, k) - h stands for the x coordinate and k stands for the y coordinate. The vertex is the center of your parabola - the highest or lowest point, the vertex or the valley, of a graph in the form of a "U" or vice versa.Being able to determine the top of a parabola is an essential part of being able to draw a correct graph - often determining the top of a parabola is part of a math problem at school.
   • In Example 1, the top of the graph is (-4.7). Draw the point on your graph and make sure you name the coordinates correctly.
   • In example 2, the top is (5.12). So from the point (0,0) you go 5 places to the right and then up 12.
6. If necessary, draw the symmetry axis of the parabola. The symmetry axis of a parabola is the line that intersects the figure in the middle, dividing it exactly in half. One side of the graph is mirrored along this line in the other side of the graph. In quadratic equations of either ax + bx + c or a (x - h) + k, this axis is the line parallel to the y axis passing through the apex of the parabola.
   • In the case of example 1, the axis of symmetry is the line parallel to the y axis and passes through the point (-4,7). Although it is not part of the parabola itself, highlighting this guideline lightly can show you how symmetrical the parabola curve is.
7. Determine the direction of the parabola. After you have found out what the top of the parabola is, it is necessary to know whether you are dealing with a mountain or a valley parabola, i.e. whether the opening is at the bottom or at the top. Fortunately, this is very easy. If "a" is positive, you are dealing with a valley parabola; if "a" is negative it is a mountain parabola (with the opening at the bottom)
   • In example 1 we are dealing with the function (f (x) = 2x + 16x + 39), so this is a valley parabola, because a = 2 (positive).
   • In example 2 we are dealing with the function f (x) = 4 (x - 5) + 12), and this is also a valley parabola because a = 4 (positive).
8. Determine if necessary the intersection points of the parabola. Often when a math problem is asked to give the intersections of the parabola with the x-axis (these are "zero", a or two points where the parabola intersects or hits the x axis). Even if not requested, these points are very important to be able to draw an accurate graph. But not all parabolas have an intersection with the x-axis. If you are dealing with a valley parabola and the valley point is above the x-axis or, in the case of a mountain parabola, just below the x-axis, then there are simply no intersection points to be found. If so, use one of the following methods:
   • Determine that f (x) = 0 and solve the equation. This method may work for simple quadratic equations, especially in the vertex form, but you will find that this becomes increasingly difficult as the functions become more complex. Below are a few examples.
     • f (x) = 4 (x - 12)
     • 0 = 4 (x - 12) - 4
     • 4 = 4 (x - 12)
     • 1 = (x - 12)
     • SqRt (1) = (x - 12)
     • +/- 1 = x -12. x = 11 and 13 are the intersection points with the x-axis of the parabola.
   • Factor the equation. Some equations in the form ax + bx + c can be easily rewritten as (dx + e) ​​(fx + g), where dx × fx = ax, (dx × g + fx × e) = bx, and e × g = c. In this case, the x intersections are the values ​​of x where each term within the parentheses becomes equal to 0. For example:
     • x + 2x + 1
     • = (x + 1) (x + 1)
     • In this case, the intersection point is -1 because, entered in both factors, this yields zero.
   • Use the abc formula. If it is not easy to figure out the intersections, or factorize the equation, use the "abc formula" specifically for this purpose. Assume an equation in the form ax + bx + c. Then enter the values ​​of a, b, and c, in the formula x = (-b +/- SqRt (b - 4ac)) / 2a. Note that this often gives you two answers for x, which is fine - that just means your parabola has two intersections with the x axis. Here's an example:
     • Enter -5x + 1x + 10 in the equation in the following way:
     • x = (-1 +/- SqRt (1 - 4 (-5) (10))) / 2 (-5)
     • x = (-1 +/- SqRt (1 + 200)) / - 10
     • x = (-1 +/- SqRt (201)) / - 10
     • x = (-1 +/- 14.18) / - 10
     • x = (13.18 / -10) and (-15.18 / -10). The intersection points of the parabola with the x axis are approximately x = -1,318 and 1,518
     • As in example 1 with the equation 2x + 16x + 39, this will look like this:
     • x = (-16 +/- SqRt (16 - 4 (2) (39))) / 2 (2)
     • x = (-16 +/- SqRt (256 - 312)) / 4
     • x = (-16 +/- SqRt (-56) / - 10
     • Since it is not possible to find the square root of a negative number, we know that there are no intersections with the x axis for this particular parabola.
9. If necessary, determine the intersection of the parabola with the y-axis. It is often not necessary, but sometimes required to find this intersection, for example for a math problem. This is fairly easy - set the value of x to 0 and solve the equation for f (x) or y, which gives you the y value of the point where the parabola intersects with the y axis. The difference with the intersection points through the x-axis is that at the y-axis there is always only one intersection point. Note - with standard equations, the y-intersection point is y = c.
   • For example, we know that our quadratic equation 2x + 16x + 39 has an intersection point y = 39, but we can also find this as follows:
     • f (x) = 2x + 16x + 39
     • f (x) = 2 (0) + 16 (0) + 39
     • f (x) = 39. The intersection of the parabola with the y-axis: y = 39. As indicated above, we can easily read the intersection point because y = c.
   • The equation 4 (x - 5) + 12 has an intersection with the y-axis which can be found as follows:
     • f (x) = 4 (x - 5) + 12
     • f (x) = 4 (0 - 5) + 12
     • f (x) = 4 (-5) + 12
     • f (x) = 4 (25) + 12
     • f (x) = 112. The intersection with the y-axis: y = 112.
10. If you think this is necessary, first draw extra points and then the entire graph. You should now have a top or a valley, a direction, intersection points with the x-axis and possibly with the y-axis of your equation. From this point on you can try to draw the parabola using these points or you can try to find more points to make the graph more accurate. The easiest way to do this is simply to enter a number of x values, which will return a number of y values. You will often be asked (by the teacher) to calculate a number of points before you can start drawing the parabola.
    • Let's take another look at the equation x + 2x + 1. We already know that the only intersection with the x axis is (-1,0). Since it only touches the x axis at this point, we can deduce that the top of the graph is equal to this point. So far we only have one point of this parabola - not nearly enough to draw a graph. Let's find a few more points to make sure we have more values.
      • Let's try to find the y values ​​that correspond to the following x values: 0, 1, -2, and -3.
      • x = 0: f (x) = (0) + 2 (0) + 1 = 1. Then the point (0,1).
      • x = 1: f (x) = (1) + 2 (1) + 1 = 4. Then the point (1,4).
      • x = -2: f (x) = (-2) + 2 (-2) + 1 = 1. Then the point (-2,1).
      • x = -3: f (x) = (-3) + 2 (-3) + 1 = 4. Then the point (-3,4).
      • Place these points in the graph and draw your parabola. Note that the parabola is completely symmetrical - if you know the points on one side of the graph, you can usually save yourself a lot of work by using these points to find the points on the other side of the symmetry axis .

Tips

• If necessary, round numbers or use fractions. This can help to display a graph correctly.
• Note that if, for the function f (x) = ax + bx + c, b or c are equal to zero, those terms will disappear. For example, 12x + 0x + 6 becomes equal to 12x + 6 because 0x is equal to 0.